Contents
The majority of the electromechanical systems that we will study have coupling between a mechanical system and a magnetic field. This text does not discuss the physics of this coupling, but makes use of some of the results. If you want to know more about the coupling between magnetic fields and conductors you should consult an introductory physics book. The fundamentals are not very important to us, but we will use two well know relationships, the Lorentz force and the induced voltage. However, we start with a review of the right hand rule and the depiction of vector fields.
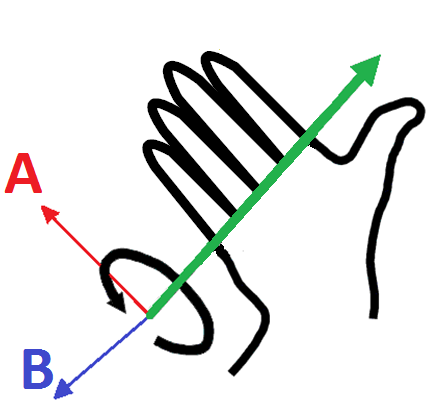
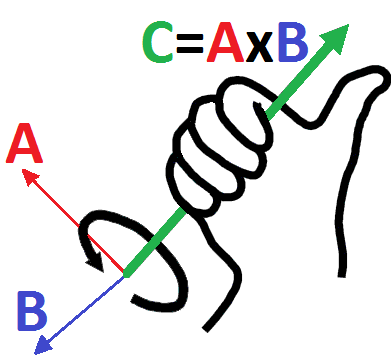
The "right hand rule" is used to find the direction of the cross product of two vectors. Given two vectors, A and B, we find the direction of the cross product, C=AxB by using the right hand rule. One method for finding the direction of C is to start with the fingers of your right hand pointing in the direction of A, then curl you fingers in the direction of B. If you do so, your thumb will be pointing in the direction of C.


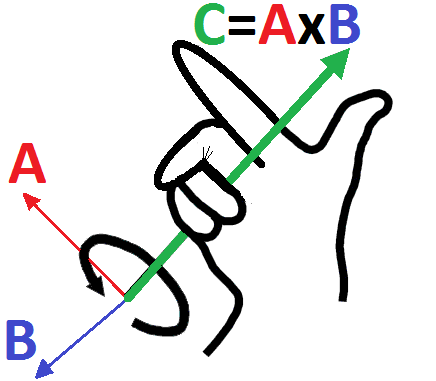
Another method is to point your index finger in the direction of A, point your middle finger in the direction of B, then your thumb is pointing in the direction of C.
We will need to depict vector fields (typically magnetic fields), but the will always be uniform. The field may be drawn from several different perspectives:
| Field from left to right (arrows represent field lines) |
 |
| Field coming out of the page (image seeing the tips of the arrows coming towards you) |
 |
| Field going in to the page (image seeing back end of arrows) |
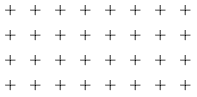 |
The Lorentz Force law states that a charge moving in a uniform magnetic field experiences a force given by
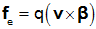
where fm is the force, q is the charge that is moving, v is velocity and β is the magnetic field strength. If the velocity of the charges is perpendicular to the magnetic fiels (i.e., v is perpendicular to β) we can write an equation for the magnitude of the force as
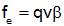
with the direction given by vxβ. If the wire has length ℓ within the magnetic field and it takes a time Δt to move through the wire, then v=ℓ/Δt, so
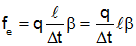
The term q/Δt is just the charge per unit time (or current) flowing through the wire. Therefore if a length of wire, ℓ, is within a uniform magnetic field, β, and has a current, i, through the wire, then the wire experiences a force with magnitude
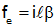
and direction given by
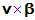
where the vector v is in the direction of the current flow.
This is demonstrated in the image below (v is up and β is into the page so
vxβ is to the left).
Important note: v is in the direction of the current; it
is not determined by the direction in which the wire is moving.
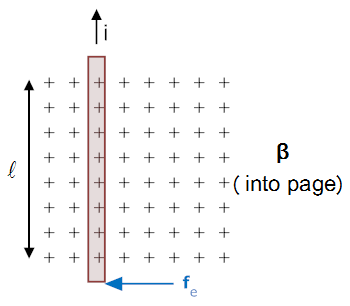
Faraday's law states that the voltage induced across a conducting bar of length ℓ moving at a velocity, v, in a uniform magnetic field, β, develops a voltage, em, given by


In the cases we will consider, v and β will be perpendicular, and the vector vxβ will be parallel to dℓ, so

with the polarity of the voltage given by vxβ. If vxβ points upward, the top part of the wire is defined to be the positive direction for the voltage. In the image above the velocity is to the right, the field is into the page, so vxβ points up, so the top of the wire is defined as positive.
Induced ForceA wire with current, i, that is perpendicular to a uniform magnetic field, xxbeta;, experiences a force with magnitude
(where ℓ is the lenght of wire in the field). The direction of the force is given by
where the vector v is in the direction of the current. |
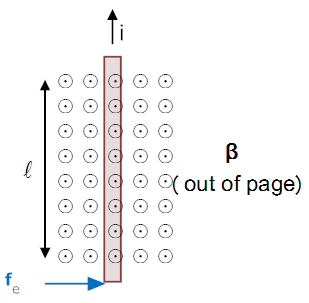 |
Induced VoltageA moving with velocity v perpendicular to a uniform magnetic field, xxbeta;, generates a voltage of magnitude
(where ℓ is the lenght of wire in the field). The positive side of the voltage on the wire is defined by
|
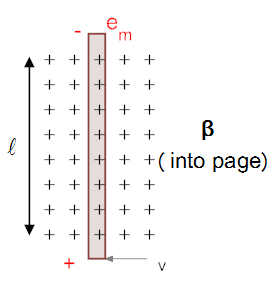 |
© Copyright 2005 to 2019 Erik Cheever This page may be freely used for educational purposes.
Erik Cheever Department of Engineering Swarthmore College