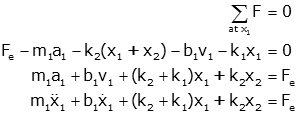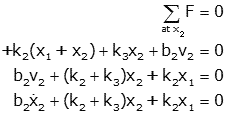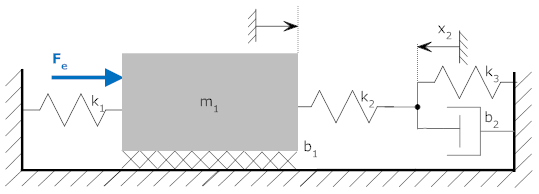
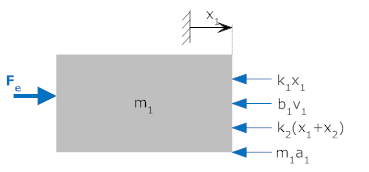
Rework of "Example: More than one unknown position" with different positions defined. 

Example: Rework of "Example: More than one unknown position" with direction of x2 reversed

Differences between this example and the original are given as bold italics.

 

The drawing above shows a system with two unknown positions, x1 and x2.  In this case we need to make two free body diagrams, one for x1, and one for x2.

Free body diagram at x1   Free body diagram at x2

There are 5 forces acting:

  1. The external force, Fe, to the right.
  2. The force due to k1.
    • If x1 increases, k1 elongates which causes a force at x1 to the left.
    • The resulting force is k1·x1 to the left.
  3. The force due to b1.
    • If x1 moves to the right  (i.e., the positive direction), the friction b1 causes a force (at x1) that is to the left.
    • The resulting force is b1·v1 to the left.
  4. The force due to k2.
    • If x1 increases, k2 compresses which causes a force at x1 to the left.
    • If x2 increases, k2 compresses which causes a force at x1 to the left.
    • The resulting force is k2·(x1+x2) to the left (or k2·(x2+x1) to the right)
  5. The force due to m1 (don't forget this - the inertial force!).
    • The resulting force is m1·a1 to the left (the inertial force is always in the opposite direction from the define positive direction).
  

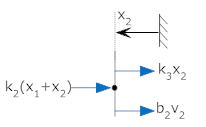
There are 3 forces acting:

  1. The force due to k2.
    • If x1 increases (to the right), this compresses the spring and causes a force to the right at x2.
    • If x2 increases (to the left), this compresses the spring and causes a force pulling x2 to the right.
    • The resulting force is k2·(x1+x2) to the right.
  2. The force due to k3.
    • If x2 increases, this elongates the spring and causes a force to the right.
    • The resulting force is k3·x2 to the right.
  3. The force due to b2.
    • If x2 moves to the left (i.e., the positive direction), this elongates the dashpot and causes a force to the right.
    • The resulting force is b2·v2 to the left.
     
     

← Back ←

References

© Copyright 2005 to 2019 Erik Cheever    This page may be freely used for educational purposes.

Erik Cheever       Department of Engineering         Swarthmore College